Physics-Based Multiscale Continuum-Discrete Deformable Terrain Model for Off-Road Mobility Simulation

Project began in 2017 and is ongoing.

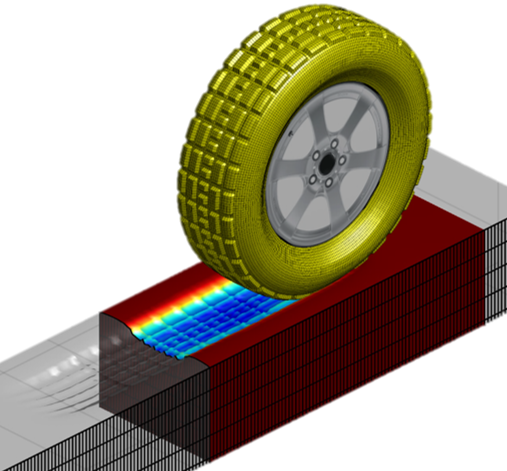
The primary objective of this project is to develop a new multiscale continuum-discrete deformable terrain dynamics model suited for physics-based off-road mobility simulation. To this end, FE-DE (finite element - discrete element) multiscale computational soil models for tire-terrain interaction will be developed that can be fully integrated into a multibody dynamics off-road mobility simulation and compared with single-scale FE and DE models to quantitatively assess the multiscale simulation capabilities for off-road mobility performance prediction.

Of itself, this comparative performance evaluation will comprise a comprehensive effort to facilitate better understanding of the multiscale approach for tire-terrain interaction problems based on a unified performance metric. This evaluation will also provide important information on the appropriate selection of simulation tools and models for operational planning for GVSC.

A high-fidelity computational model for deformable terrains is essential for physics-based off-road mobility simulation in achieving accurate mobility performance prediction as well as reliable operational planning. Granular terrain dynamics is complex, involving highly nonlinear anisotropy of strength, strain localization, and solid-fluid state transition. Whereas the use of empirical or simplified models can lead to unrealistic prediction of mobility performance, physics-based modeling becomes critically important in predicting such nonlinear deformable soil behavior.

GVSC has taken a leading role in developing the Next Generation NATO Reference Mobility Model (NG-NRMM) to replace existing empirical simulation models with physics-based models to enhance off-road mobility performance prediction capabilities. High-fidelity terrain dynamics model is one of the priority objectives in the NG-NRMM. In this proposed research, we will develop a new physics-based multiscale continuum-discrete deformable terrain model suited for physics-based off-road mobility simulation.

Publications:

1. Yamashita, H., Chen, G., Brauchler, A., Ruan, Y., Jayakumar, P. and Sugiyama, H., 2018, “Computer Implementation of Hierarchical FE-DE Multiscale Approach For Modeling Deformable Soil in Multibody Dynamics Simulation”, Proceedings of ASME International Conference on Multibody Systems, Nonlinear Dynamics, and Control (ASME DETC2018-86110), Quebec City, Quebec, Canada.
2. Yamashita, H., Jayakumar, P., Alsaleh, M. and Sugiyama, H., 2017, “Physics-Based Deformable Tire-Soil Interaction Model for Off-Road Mobility Simulation and Experimental Validation”, ASME Journal of Computational and Nonlinear Dynamics, vol. 13, pp. 021002-1-15.

Publications from Prior Work:

• Yamashita, H., Jayakumar, P., Alsaleh, M. and Sugiyama, H., 2018, “Physics-Based Deformable Tire-Soil Interaction Model for Off-Road Mobility Simulation and Experimental Validation”, ASME Journal of Computational and Nonlinear Dynamics, doi: 10.1115/1.4037994.
• Yamashita, H., Jayakumar, P. and Sugiyama, H., 2016, “Physics-Based Flexible Tire Model Integrated with LuGre Tire Friction for Transient Braking and Cornering Analysis”, ASME Journal of Computational and Nonlinear Dynamics, vol. 11, pp. 031017-1-17.
• Recuero, A., Serban, R., Peterson, B.*, Sugiyama, H., Jayakumar, P. and Negrut, D., 2017, “High-Fidelity Approach for Vehicle Mobility Simulation: Nonlinear Finite Element Tires Operating on Granular Material”, Journal of Terramechanics, vol. 72, pp. 39-54.
• Yamashita, H., Valkeapää, A., Jayakumar, P. and Sugiyama, H., 2015, “Continuum Mechanics Based Bi- Linear Shear Deformable Shell Element Using Absolute Nodal Coordinate Formulation”, ASME Journal of Computational and Nonlinear Dynamics, vol. 10, pp. 051012-1-9.